Pillow-Problems: Problem #15

Problem #14 | Problem #16
For more information on this collection, see Pillow-Problems by Charles L. Dodgson (Lewis Carroll).

Problem:

If a Figure be such that the opposite angles of every inscribed Tetragon are supplementary: the figure is a Circle.

Solution:

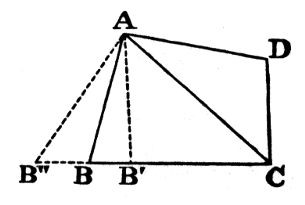
Let ABCD be an inscribed Tetragon. Join AC: and about Triangle ACD describe a Circle.

Now, if this Circle does not pass through B, let it cut CB, or CB produced, in B′ or Bʺ. Join AB′, ABʺ.

Then ∠ AB′C, or ∠ABʺC, is supplementary to ∠ADC;

∴ it = ∠ABC, which is absurd;

∴ this Circle does pass through B.

The same thing may be proved for any other Point on that portion, of the perimeter of the given Figure, which lies on the same side of AC as the Point D.

Similarly for the other portion.

Hence the Figure is a Circle.

Q.E.D.