Pillow-Problems: Problem #40

Problem #39 | Problem #41
For more information on this collection, see Pillow-Problems by Charles L. Dodgson (Lewis Carroll).

Problem:

In a given Triangle, whose base-angles are acute, draw two lines, at right angles to the base, and together equal to the line drawn, from the vertex, at right angles to the base, and such that

(1) they are equidistant from the line drawn from the vertex;

(2) they are equidistant from the ends of the base.

Solution:

(1) Let ABC be the given Triangle, and AD the line from the vertex.

From D draw DE, DF, parallel to the sides; and from E and F draw EG, FH, ⟂ BC.

Then Triangles FBD, EDC, are similar to ABC;

∴ FH : AD :: BD : BC,

and EG : AD :: DC : BC;

∴ (FH + EG) : AD :: BC : BC;

∴ FH + EG = AD.

Also, ∵ Triangles AED, AFD, are equal and on the same base AD,

∴ their altitudes are equal; i.e. DH = DG.

Q.E.F.

(2) Let ABC be the given Triangle, and AD the line from the vertex.

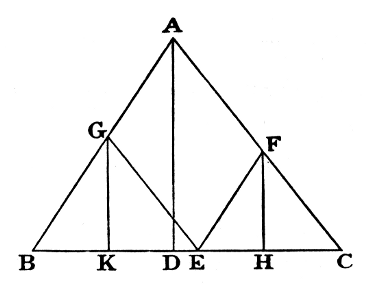
Make CE = BD; from E draw EF, EG, parallel to the sides; and from F, G, draw FH, GT ⟂ BC.

Then Triangles GBE, FEC, are similar to ABC;

∴ GK : AD :: BE : BC,

and FH : AD :: EC : BC;

∴ (GK + FH) : AD :: BC : BC;

∴ GK + FH = AD.

Also BK : BE :: BD : BC;

∴   BK : DC :: EC : BC;

          :: HC : DC;

∴ BK = HC.

Q.E.F.