Solutions for Practice Test 2, The Official SAT Study Guide, Section 8

Here are solutions for section 8 of the second practice test in The Official SAT Study Guide, second edition, found on pages 481–486. The following solutions illustrate faster, less formal methods that may work better than formal methods on a fast-paced test such as the SAT. To learn more about these methods, see my e-book Succeeding in SAT Math or the SAT math tips page.

1. 15 minutes after the show begins, 15⁄90 of it is complete. 15⁄90 = 1⁄6. Select (B) 1⁄6.
2. Solution 1: We can assume that the diagram is drawn to scale. Just by glancing at the diagram, you can tell that the answer must be either (B) HK or (D) JK. Using your finger or your pencil, carefully measure these two. You will find that the longest side is (D) JK. Select that answer.

   Solution 2:

   • You may know that, in a triangle, the longest side is opposite the largest angle. We can assume that the diagram is drawn to scale.
   • Use process of elimination: In triangle JHK, angle H appears to be the largest angle, so JK is the longest side; hence, (A) HJ, (B) HK are not the right answers; we can also eliminate (C) HL because it is obviously smaller than HK. Now, in triangle JLK, the largest angle appears to be L and so the longest side is JK. We can eliminate (E) JL, so the answer is (D) JK.

   Solution 3: Looking at the diagram, it's obvious that the answer must be either (B) HK or (D) JK. Both lines form part of triangle JHK. The largest angle in this triangle is angle H. Since the largest angle is opposite the largest side, JK must be larger than HK. Select (D) JK.

   Solution 4:

   • Draw a diagram: The diagram is given. Mark on it that ∠JHK is a right angle.
   • In a right triangle, the hypotenuse is the longest side. So, in triangle JHL, the longest side is JL. In triangle JHK, the longest side is JK. Based on this, we can eliminate (A), (B), and (C).
   • Using the Pythagorean theorem (given in the Reference information at the top of the page), we know that JL² = JH² + HL². Similarly, JK² = JH² + HK². Additionally:
     HK > HL
     ∴ JH² + HK² > JH² + HK²
     ∴JK > JL
     Select (D) JK.
3. Solution 1: Look for a pattern: Each number in the f(n) column increases by 6 for every box you go to the right. So, p must equal 19 + 6 = 25. Select (C) 25.

   Solution 2:

   • Estimate the answer: Since the table defines a linear function, p must be halfway between 19 and 31. This appears to be 25.
   • Look at the answer choices: 25 is one of the choices.
   • Guess and check: Check whether 25 is the correct answer. 25 is 6 away from 19, and it is also 6 away from 21. Therefore, the answer is (C) 25.
4. Solution 1: Convert the sentence into an equation as follows:

   5 years	5
   less than	[subtract the previous term from the following term]
   twice as long as	2 ×
   Maly has	n

   The result is 2n − 5. Select (C) 2n − 5.

   Solution 2:

   • Try a special case: Say that Maly has built houses for, say, 3 years (so, n = 3). then, twice as long as Maly has built houses for is 6 years, and so Charlie has built houses for 5 years less than 6 years = 1 year.
   • Look at the answer choices: Evaluate each answer to see which evaluates to 3:

     (A)	3 − 5 = − 2
     (B)	3 + 5 = 8
     (C)	2(3) − 5 = 1
     (D)	2(3) + 5 = 11
     (E)	5 − 2(3) = −1

     The only answer evaluating to 1 is (C) 3. Select that answer.
5. • Estimate the answer: We can assume the diagram is drawn to scale. So, we can eyeball the ∠CPD. It definitely appears to be less than 80°. Looking at the 45° angle in the Reference Information, it appears to be slightly larger than that, maybe 50° or 60°.
   • Look at the answer choices: Based on our estimate, we can eliminate (A) 40°, (D) 70°, and (E) 80°. Two answers, (B) 50° and (C) 60°, remain.
   • The angles BPA and BPD are supplementary (add up to 180°). Since ∠BPA = 80°, ∠BPD = 100°.
   • Since PC bisects ∠BPD, ∠CPD = ½(∠BPD) = ½(100°) = 50°. Select (B) 50°.
6. Try a special case: Let x = 1. Then, the next odd number greater than x is 3. Next, evaluate each answer choice to see which are equal to 3:

   (A)	1 − 1 = 0
   (B)	1 + 1 = 2
   (C)	1 + 2 = 3
   (D)	1 + 3 = 4
   (E)	2(1) − 1 = 1

   The only answer yielding 3 when x = 1 is (C) x + 2. Select that answer.

   Note: If you chose a different number as a special case, such as 3, then both (C) and (E) might match. In that case, try a different special case,

7. • Draw a diagram: The graph is already drawn, but label a and b on the axes.
   • Estimate the answer: Based on the labelling, the y-coordinate of T must be b.
   • Look at the answer choices: The only answer with a y-coordinate of b is (A) (−a, b). Select that answer.
8. • Read the question carefully and determine what it is asking: There are four times as many (blue glass beads + red glass beads) as wood beads. There are three time as many red glass beads as blue glass beads. There are 12 red glass beads. We are asked for the total number of beads in the box.
   • Estimate the answer: Based on the information given, there must be more red glass beads than blue glass beads, and more red glass beads than wood beads. So, the total number of beads must be less than 3 × 12 = 36; perhaps somewhere between 16 and 30 might be a good estimate.
   • Look at the answer choices: The only answer choice in range of our estimate is (A) 20. Select that answer.
9. • Draw a diagram: On the diagram given in the question, draw the reflection of the graph in the x-axis.
   • Look at the answer choices: The answer choice corresponding to the reflection you just drew is (A). Select that answer.
10. • First, expand the two equations:
      x² + 2xy + y² = 100 (1)
      x² − 2xy + y² = 16 (2)
    • We are looking for the value of xy, so we want to get it by itself. We can do this if we subtract (2) from (1):
      4xy = 84
      xy = 21
      Select (C) 21.
    Solution 2:
    • Look at the answer choices: All of the answer choices are positive. This means that either both x and y are positive, or they are both negative.
    • Make an assumption: Assume that both x and y are positive integers. If we work the problem and find it doesn't work out, we can make a different assumption. So, taking the square root of the first equation, we get x + y = 10.
    • Guess and check: There are several possible solutions for the first equation (e.g. x = 9, y = 1, x = 7, y = 3, x = 5, y = 5, among others). However, the answer must satisfy the following:
      • It must be one of the listed answers (6, 10, 21, 25, 29).
      • It must make (x − y)² = 16 true.
      Trying a few answers, you should find one of two that work: x = 7, y = 3, or x = 3, y = 7. Either way, xy = 21. Select (C) 21.
11. • Look at the answer choices: There are a variety of choices. Some include x = 1; others don't. Some include x = 0; others don't. Some, but not others, include larger values or smaller values. It may be worthwhile to try special cases to eliminate answers.
    • Try a special case: Say that x = 1. Our inequality becomes:
      −1 ≤ 4(1) − 5
      −1 ≤ −1
      which is true. (B), (C), and (D) do not include 1 in the given range, however, so they can be eliminated.
    • Try another special case: Say that x = 0. Our inequality becomes:
      −1 ≤ 4(0) − 5
      − 1 ≤ −5
      which is false. Since (E) includes 0 in its range, it can be eliminated, and the correct answer is (A).
12. There are a few ways to look at this question, which doesn't require any calculation, but it requires some insight. A circle has rotational symmetry of infinite order. So, you could rotate the given figure any number of degrees and still have the same circle, but with two differently-aligned rectangles in it. So, there must be an infinite number of possibilities. Select (E) More than four.
13. • Try a special case: Say that n = 1. Then k = 2¹ + 2² = 6. Now, 2^{n + 2} = 2³ = 8.
    • Look at the answer choices: See which answer choices evaluate to 8 when k = 6:

      (A)	(6 − 1)/2 = 5/2
      (B)	4(6)/3 = 8
      (C)	2(6) = 12
      (D)	2(6) + 1 = 13
      (E)	6² = 36

      The only one that evaluates to 8 is (B) 4k/3. Select that answer.
14. This question, while not too difficult, can trip you up if you don't realize there are two possibilities for the length of BC.
    Solution 1:
    • If the triangle is isosceles, then either BC = AB or BC = AC.
    • Either way, AB is a longest side of the triangle, and AC is a shortest side of the triangle.
    • In a triangle, the largest angle is opposite the longest side, and the shortest angle opposite the shortest side. So, z° is the largest angle and y° the smallest angle. So, they can't be equal. Select (E) y = z.
    Solution 2:
    • Draw a diagram: The figure given is not drawn to scale, so draw a figure that matches the given information. Note that there are two possibilities, both of which are shown below:
      
    • Use process of elimination: Looking at the diagrams, BC could equals AB in the first diagram and AC in the second diagram, so (A) and (B) are incorrect. In the second diagram x = y, so (C) is incorrect. x = z in the first diagram, so (D) is incorrect. Select (E) y = z.
15. • Estimate the answer: Tom paid $240 for the entire trip. He paid 20% of that for the hotel room. If he had to pay for the entire hotel room, that would cost 80% of his $240. A good estimate for 80% of $240 might be around $200.
    • Look at the answer choices: The only choice anywhere near $200 is (D) $192. Select that answer.
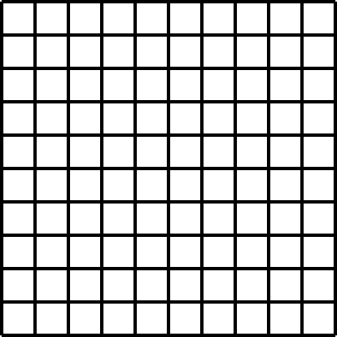
16. Solution 1:
    • Draw a diagram and look for a pattern: Draw a few smaller grids and count the number of squares along the boundary of the gameboard:

      4	8	12	16

      So, it looks like the number of squares is always a multiple of 4.
    • Look at the answer choices: The only multiple of 4 in the answer choices is (E) 52. Select that answer.
    Solution 2:
    • Draw a diagram: Draw a game board:
      
    • Now, the number of squares on the left edge is n. The number of squares on the right edge is n. The number of squares on the top edge that have not yet been counted is n − 2 (the corner squares have already been counted. The number of squares on the bottom edge that have not already been counted is n − 2. In total, there are:
      n + n + n − 2 + n − 2
      = 4n − 4
      = 4(n − 1)
      So, the number will always be a multiple of 4.
    • Look at the answer choices: The only multiple of 4 in the answer choices is (E) 52. Select that answer.